In last 1 decade, options as an instrument has grown high popularity in Indian derivatives market segment as they have great leverage power, less risk and higher potential returns. Options with these advantages have attracted investor to invest in available options (index options and stock options) in the financial markets. But another facet of the options is that these are very sophisticated instruments and many brokers in the market are not well trained and this lack of information made option risky investment for individual investors. To make option fruitful it is necessary to understand how options are valued and what will be the impact of change in the price of underlying value or other factors.
How options are valued?
Before starting trading on options it is crucial to understand option pricing and factors influencing the value of options. Value of option is derived from its underlying asset (stock, index, commodity, etc). The value of option is known as premium. An option’s value is based on a combination of strike price, time to expiration, interest rates, dividends, and volatility around the fair value asset price. The price of a call option rises with rise in spot price as due to rise in prices the option becomes more likely to exercise. It however falls with the rise in strike price. The opposite is true for the price of put options. The rise in volatility levels of the stock price however leads to increase in price of both call and put options. The option price is higher for an option which has a longer period to expire. Option prices tend to fall as contracts are close to expiry. This is because longer the term of an option higher is the likelihood or probability that it would be exercised. The rise in risk free rate tends to increase the value of call options and decrease the value of put options. Similarly price of a call option is negatively related with size of anticipated dividends. Price of put option positively related with size of anticipated dividends.
Black & Scholes Model
In 1973 Fisher Black and Myron Scholes developed a mathematical model and published in their paper “The pricing of options and corporate liabilities”. Later on Robert Merton expand the mathematical equation of option pricing. In 1997 Scholes and Merton received noble prize for Black & Scholes Model. Black & Scholes model is the most used model to calculate the value of option, which takes underlying value, strike price, volatility, interest rate and time to expiration into consideration.
B&S Model Equation:
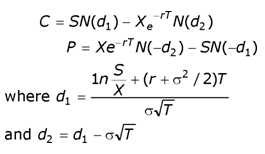
In the above mentioned model, ‘C’ represents the equation for call option and ‘P’ represents the put option. The model is divided into two parts: the 1st part, SN(d1), multiplies the price by the change in the call premium in relation to a change in the underlying price. This part of the formula shows the expected benefit of purchasing the underlying outright. The 2nd part, N(d2)Ke-rt provides the current value of paying the exercise price upon expiration.
Black & Scholes option pricing model works on the basis of following assumptions:
-
There is no arbitrage opportunity.
-
It is possible to borrow and lend cash at a known constant risk-free interest rate.
-
It is possible to buy and sell any amount, even fractional, of stock.
-
The above transactions do not incur any fees or costs.
-
The stock price follows a geometric Brownian motion with constant drift and volatility.
-
The underlying security does not pay a dividend.
Option Greeks
As we discussed above that option’s price can be influenced by number of factors. To understand the impact of these factors it is essential to know briefly about option greeks. Option greeks measures the sensitivity of an option’s price to change in underlying price, strike price, volatility, time to expiry and short term interest rate. Option Greeks allow option traders to calculate changes in the value of the option contracts in their portfolio with respect to changes in the factors that affects the value of options. Traders basically use option greeks to hedge their position on the risk associated with their portfolio or construct position according to risk and reward profiles. Professional option traders mostly use the option greeks such as Delta, Gamma, Vega, Theta and Rho to strategize their option trades.
Though, all these factors have been discussed in detail in the NISM study material which has been prescribed for the certification of NISM Series VIII: Equity Derivatives Examination, yet we are providing hereunder the snapshots of these option greeks.
Delta: A delta of an option measures the change in option price with respect to the change in the price of underlying asset. It measures the increase or decrease in the option price in response to a 1 point movement of the underlying asset. The value of delta lies between 0 to 1 in case of call option and 0 to -1 in case of put option. Deep in-the-money options have delta value of 1, At-the-money options have 0.5 and Far out-of-the-money options have 0 value. Call options have positive delta because call option premium is positively related to the price of underlying; call premium rises with the increase in the price of the underlying, whereas, put options have negative delta because put premium have negative relationship with the price of underlying asset.
Gamma: It measures the change in the delta of an option with respect to the change in the price of underlying asset. It is also known as “first derivative of delta”. It measures the change in the value of delta with respect to a 1 point movement of the underlying asset. Gamma is smallest for deep out-of-the-money and deep in-the money options. It is highest when the option gets near the money. It is positive for long options and negative for short options.
Vega: It reflects how much an option’s premium changes with each 1-percent change in implied volatility. Changes in volatility affect call and put options in a similar way. An increase in volatility will increase the prices of all the options in an asset, and vice versa. Short options will have negative vega as it has inverse relationship with volatility; short position on option will gain if implied volatility falls and vice versa. Vega can increase from quick moves of the underlying, especially if there is a big drop in the stock market. It falls as the option gets closer to expiration.
Theta: A measure of an option's premium sensitivity to time decay. The theta value indicates how much value a stock option's price will diminish per day with all other factors being constant. Theta will increase sharply in the last few weeks of trading and can severely undermine a long option holder's position, especially if implied volatility is on the decline at the same time. It is typically highest for at-the-money options.
Rho: It measures the change in option premium relative to the change in the risk-free interest rate. It estimates how much the option’s price will change when interest rates changes by 1%. Positive rho means the option price increases when the interest rate increases and decreases when the interest rate decreases. Negative rho means the option price decreases when the interest rate increases, and increases when the interest rate decreases. Increase in the interest rate will increase the value of call option and decrease the value of put options.